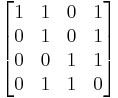Bondy's theorem

In mathematics, Bondy's theorem is a theorem in combinatorics that appeared in a 1972 article by John Adrian Bondy.[1] The theorem is as follows:

Let X be a set with n elements and let A1, A2, ..., An be distinct subsets of X. Then there exists a subset S of X with n − 1 elements such that the sets Ai ∩ S are all distinct.

In other words, if we have a 0-1 matrix with n rows and n columns such that each row is distinct, we can remove one column such that the rows of the resulting n × (n − 1) matrix are distinct.[2][3]

From the perspective of computational learning theory, this can be rephrased as follows:

Let C be a concept class over a finite domain X. Then there exists a subset S of X with the size at most |C| − 1 such that S is a witness set for every concept in C.

This implies that every finite concept class C has its teaching dimension bounded by |C| − 1.

Example

Consider the 4 × 4 matrix

\begin{bmatrix} 1&1&0&1\\ 0&1&0&1\\ 0&0&1&1\\ 0&1&1&0 \end{bmatrix}

where all rows are pairwise distinct. If we delete, for example, the first column, the resulting matrix

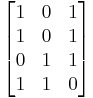
\begin{bmatrix} 1&0&1\\ 1&0&1\\ 0&1&1\\ 1&1&0 \end{bmatrix}

no longer has this property: the first row is identical to the second row. Nevertheless, by Bondy's theorem we know that we can always find a column that can be deleted without introducing any identical rows. In this case, we can delete the third column: all rows of the 3 × 4 matrix

\begin{bmatrix} 1&1&1\\ 0&1&1\\ 0&0&1\\ 0&1&0 \end{bmatrix}

are distinct. Another possibility would have been deleting the fourth column.

Notes

  1. ^ Bondy, J. A. (1972), "Induced subsets", Journal of Combinatorial Theory, Series B 12: 201–202, doi:10.1016/0095-8956(72)90025-1, MR0319773 .
  2. ^ Jukna, Stasys (2001), Extremal Combinatorics with Applications in Computer Science, Springer, ISBN 9783540663133 , Section 12.1.
  3. ^ Clote, Peter; Remmel, Jeffrey B. (1995), Feasible Mathematics II, Birkhäuser, ISBN 9783764336752 , Section 4.1.